Finding Exact Values of Inverse Trigonometric Functions

Each year it seems my PreCalculus students are confused when it comes to truly understanding inverse functions, even though they have been exposed to the concepts and ideas in many courses prior to their PreCalculus year. With the introduction to Inverse Trig Functions they seem lost. I see deer in the headlights, “glazed-over” eyes, staring back at me. Can you relate to that? I often think, “Is it me, or is this just a really confusing idea for their young brains?”
How do you get your students to “see” these values occur in Quadrant I and Quadrant IV? The function is periodic, repetitive, oscillating! Am I speaking Greek to you? Seriously, people!! Don’t take me wrong! I truly care about these students. When you get lost in math class things go downhill rapidly. Students that don’t have GRIT and DETERMINATION want to shut down. I’m their biggest cheerleader. DON’T QUIT, STAY WITH ME! We can figure this out. Having made a unit circle on really cheap paper plates early in the Trigonometry Unit, we can use our Paper Plate Unit Circles for this lesson. Each time the expression is an inverse, students fold their plates to the “RESTRICTED ZONE” for the visual connection to the domain that they are allowed to explore for solutions to their question prompt.

I like to have them think along the lines that they are asking themselves to “find the angle in the restricted area where….” fill in the blank here with sine inverse, cosine inverse, etc. How do you get your students to “see” these values occur in Quadrant I and Quadrant IV? The function is periodic, repetitive, oscillating! Am I speaking Greek to you? Seriously, people!! Don’t take me wrong! I truly care about these students. When you get lost in math class things go downhill rapidly. Students that don’t have GRIT and DETERMINATION want to shut down. I’m their biggest cheerleader. DON’T QUIT, STAY WITH ME! We can figure this out. Having made a unit circle on really cheap paper plates early in the Trigonometry Unit, we can use our Paper Plate Unit Circles for this lesson. Each time the expression is an arch!

Exact Values of Inverse Trig

Finding Exact Values of Inverse Trig

Do you have any great strategies, techniques, or methods that help your students gain insight into inverse trigonometric functions? I would love to hear from you.

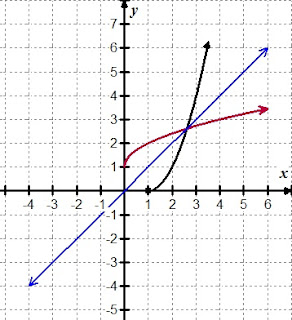
When teaching inverse trig functions, I always have my students recall their journey with inverse functions through a small discovery activity. I love using the Rule of Four approach which I fondly call G.N.A.W. (graphic, numeric, algebraic, verbal). We begin by graphing a linear function, then a quadratic function, and their inverses. Our discussion will look at the symmetry across the identify function y=x. Next, we talk about vertical line tests, horizontal line tests, and review the vocabulary for what it means to be a one-to-one function and the result of that condition. Then, we explore the algebra and everyone (well, almost everyone) can recite the procedure for how to find the inverse function with a restricted domain.
Ahh, they get it! Drum roll, please! Now let’s go look at the trigonometric functions. Can they have inverses?

Sure! Are their inverses also functions? Huh? What? I think I want to cry!

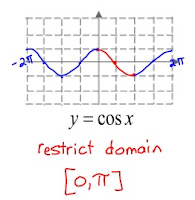
Where did I go wrong? I’ve eased them into remembering all that they had seen before, and still no connection for a large group of these learners. Finding where cosine begins to repeat for all angles from 0 to pi is easy. Ok, they got it, now!

Let’s restrict the domain and graph the inverse function in our Foldable(R).  But, then comes the sine curve. How can we find a continuous interval where the sine function takes on every value of the range from -1 to +1?

This year after teaching the lesson, my students practiced and the next day, I was ready for them. I had made an extra Interactive Notebook Foldable to help them gather their thoughts.

I also created a set of 24 task cards in a matching puzzle arrangement. I made my way around the room from group to group. The light bulb is getting brighter inside those growing brains! Yeah!

I love leading this group of students into the world of upper-level mathematics and all the thinking processes that are involved to bring them to this place in their formal education.